To the editors:
The best news about this essay appears as an editorial note right before the text begins: the author plans to write a few more such pieces! Be impatient, for they should be comparably charming, particularly the last, announced as the Standard Model. In that one, the author himself will have to join the other great scientists quoted in his first essay, standing upon the shoulders of giants, as Isaac Newton wrote in a letter to Robert Hooke.1
Under his caricature, Glashow is described as a Nobel laureate. But Sheldon (Shelly to his friends) is not just your friendly-neighborhood run-of-the-mill Nobel laureate. Here is why.
In the seventeenth century, Isaac Newton unified—i.e., found the common basic explanation for—the motions of the moon and the proverbial apple. In the nineteenth century, James Clerk Maxwell unified electricity, magnetism and light. These particular unifications are not termed grand, but they are.2 These unifications are grandiose steps forward in the progress of science. Of late, they seem to occur every century or two; the statistics are not overwhelming.
Glashow, Steven Weinberg and Abdus Salam unified electromagnetism and the weak interactions responsible for radioactivity and the functioning of the Sun. The starting point was Glashow’s thesis, the published version of which dates from 1961.3 For this unification, they shared the 1979 Physics Nobel prize. My own contribution to all this was—under strict conditions of confidentiality demanded by the then Secretary of the Nobel Committee—to photocopy Glashow’s thesis in the Harvard Physics library and to send it to Stockholm. One hopes that the current century will witness the remaining truly grand unification, that of quantum mechanics and Einstein’s theory of space-time and gravity: General Relativity.
The last-mentioned unification has been achieved by String Theory, based on mathematics of undeniable beauty.4 To be self-consistent, String Theory requires nine or ten dimensions of space. The estimated number of ways to reduce them to the observed three is greater than 10500. As Alfonso X of Castile, “King of the Three Religions,” might have observed: “Should the Almighty have consulted me before embarking on Creation, I would have recommended something simpler.”5
Unless one wishes to be a loser, it would be unreasonable to compete with Glashow on any scholarly issue from physics to crossword solving. Thus I shall only add a few somewhat random threads to the ones he wove, or did not weave.
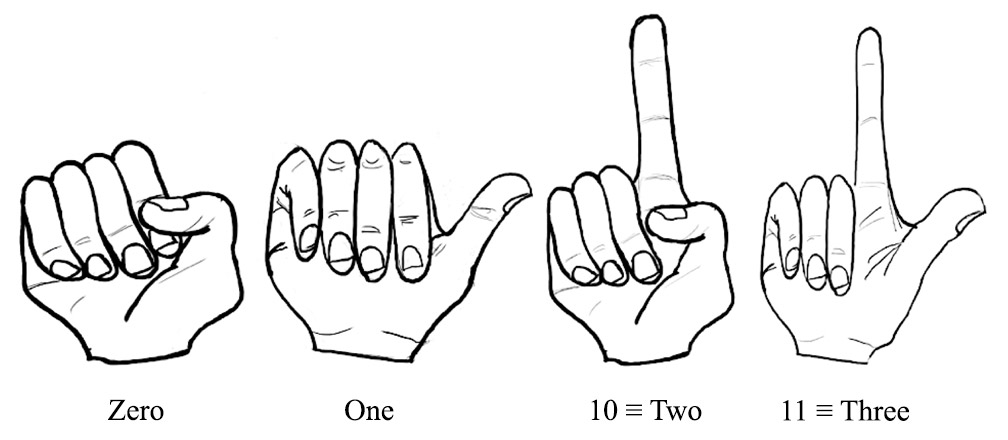
Glashow states that “Computers, using simple yes-no binary numbers, are both digital and digitless.” I would add that we are ourselves very very digit-full. Numbers on base 2 (as opposed to 10) should be promoted to common usage. Counting on your fingers is the obvious benefit. In Figure 1., one can see how to count 0, 1, 2 and 3 (4 is unbecoming, and so is 18). With one hand, one can reach up to 25 – 1 = 31 (all fingers up).
Figure 1.
From zero to 3 in “truly digital” binary.
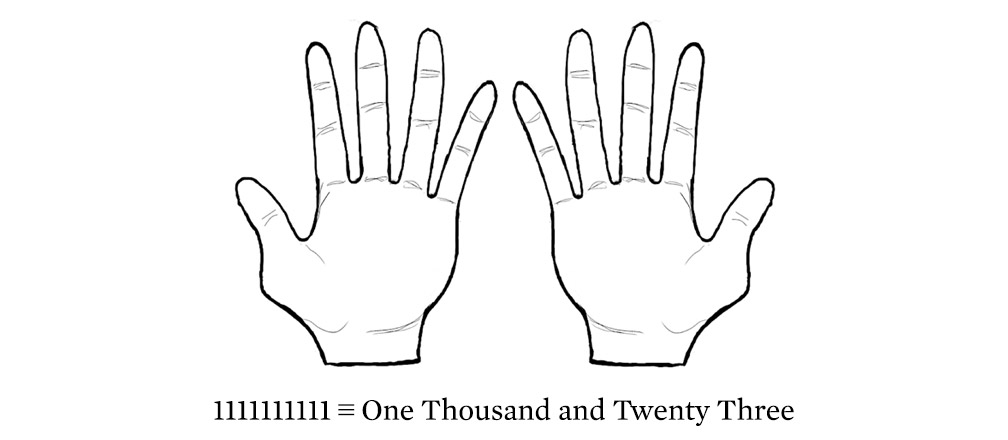
Using two hands, as in Figure 2., one can count up to 210 – 1 = 1,023. Teaching your hands to count fast in binary is an extremely good mental and physical exercise, which I would recommend mastering from an early age. In Latin, digitus means both fingers and toes. Anyone sufficiently monkey-gifted to extend the above exercises to the toes would be able to count to 220 – 1 = 1,048,575. The upper limit is not hard to master: all fingers and toes up! On the other hand, so to speak, 10101010101010101010, namely 699,050, is amongst the hardest.
Figure 2.
The decimal 1,023 in “digital” binary.
Also concerning numbers, I miss a further comment by Glashow on 0, as well as on 40. Zero is a fantastic invention and its history is fascinating. It took a long time to fully digest and utilize the notion of 0, much as it seems to be taking forever for physicists to fully understand the vacuum. Forty is pronounced sorokh in Russian. It breaks the rhythmic sequence of the ties (twenty, thirty, …, fifty…) and its etymology is worth trying to figure out. Unlike score and its foreign siblings, it probably does not take two (base-ten) Russians to count to forty on their fingers and toes.
Regarding calendars, an accidental coincidence of the Julian and Gregorian is that both Cervantes and Shakespeare died on April 23, 1616. But while it may have been the same date, it was not the same day. It now appears that Miguel de Cervantes Saavedra, to be precise to Hispanic extremes, died one true, solar day earlier than previously recorded.
In connection with the units of measurement, the absurdities to which scientists occasionally succumb are not often overemphasized. A beautiful example occurred in the days during which the meter was one ten-millionth of the length of a quarter of an Earth’s meridian. Expeditions were organized to measure the meter! Only (pseudo-) politicians can compete, extraordinarily favorably, with similar absurdities. The current definition of the meter is financially sound; it entails considerable savings in Platinum and travel.
Some sets of units are quite absurd, but tremendously charming. Perhaps Britain should consider going back to guineas, pounds, shillings, farthings, half-crowns, pennies, six- and three-pence… Fathoms, feet, octavos, imperial pints and even British thermal units each have their own charisma. If you wish to keep your brain in shape, do convert, a few times a day, umpteen miles per gallon into liters per hundred kilometers—hint: the answer is proportional to one over umpteen. At a second stage you might try the calculation in binary. Next, do it with Roman numerals to experience how welcome an occasional 0 would be.
Cuneiform may have been the first written language, but how did it sound? Georges Charpak, a physics Nobel laureate and a former member of the French Résistance who spent time in a German concentration camp, proposed a way to find out. If an ancient potter was talking or singing, while holding a pointed stone or metal object to incise a revolving vase, a circular or more complex sound track may have been engraved in it. With patience, a lot of antique pottery and a CD-like laser reader, one may succeed in listening to ancient tongues. Charpak’s brilliant idea, alas, has not found the required support, like so many other of his astute suggestions, one of them in collaboration with Glashow.6
Napoleon is quoted as saying, “L’histoire est une suite de mensonges sur lesquels on est d’accord.” The most quoted English translation—“History is the version of past events that people have decided to agree upon”—fits better my commentary on Glashow’s writing about the invention of quarks. Quarks are so fundamental in every sense that they deserve a couple of paragraphs.
Glashow’s remarks about quarks are correct, but incomplete. On December 30, 1963, an article by André Petermann was received by the journal Nuclear Physics.7 This was a few days before the dates of the papers by the other quark progenitors; it was a busy Christmas time. In his paper, Petermann discusses mesons as made of a spinor/anti-spinor pair and baryons as composed of at least three spinors—by spinors he meant novel particles with spin ½, which he did not bother to baptize; baryons and mesons are the particles whose properties the quark model elucidates. Concerning the delicate issue of the new spinor’s electrical charges, Petermann wrote: “If one wants to preserve charge conservation, which is highly desirable, the spinors must have fractional charges. This fact is unpleasant, but cannot, after all, be excluded on physical grounds.” At the time, particles with charges that were not multiples of the electron’s charge—minus 1, by convention—were anathema.
Concerning dates, it must be recalled that Gell-Mann wrote: “These ideas were developed… in March 1963; the author would like to thank Professor Robert Serber for stimulating them.”8 Serber’s recollections, if accurate, add considerable extra spice to this story.9
A main character in Glashow’s tale is Dmitri Mendeleev. His thread extends over much of the fabric of the essay’s tapestry. Like quarks, Mendeleev deserves extra commentary. He introduced the metric system in Russia, eliminating many glamorous entities, such as verstas (3,500 feet), charkas (the standard wine glass), butylkas (pretty obvious) and the irresistible sounding poods (16.3807 kg). To gauge Russian flexibility, or the difficulty of accepting an eminently sensible system of units, consider trying to impose the metric system in the Anglo-Saxon world.
Mendeleev was director of the Bureau of Weights and Measures in St. Petersburg and, as an expert on petroleum, he helped in the foundation of the first oil refinery in Russia. He is credited with the remark that “Burning petroleum (or natural gas) as a fuel would be akin to firing up a kitchen stove with bank notes.”10 One wonders what he would have said nowadays about carbon or shale oil. The cited baryons and mesons, prior to the last-century’s sixties, were not unlike the chemical elements before Meyer and Mendeleev: scores of entities lacking a systematic explanation. The quark model is analogous to the definite understanding of the Periodic Table in terms of ensembles of electrons, protons and neutrons.
Mendeleev was nominated for the Chemistry Nobel Prize in 1906 and 1907. Influenced by one of his adversaries, Svante Arrhenius, who had won this prize in 1903, the Nobel Committee did not select Mendeleev. Arrhenius was not a member of the Chemistry Committee. Yet he still succeeded in having the prize given to some of his friends (Jacobus van’t Hoff, Wilhelm Ostwald, and Theodore Richards) and denied to some of his foes, such as Mendeleev. It seems that once upon a time the Nobel Committees were not infallible. This is still the case, or so say many scientists, politicians, writers and singers… who did not get the prize.
Glashow’s intense and extremely contagious passion for knowledge permeates his essay. It feels like an oasis in these arid days.
Álvaro De Rújula
Sheldon Lee Glashow replies:
The basis for East Asian tetraphobia becomes a most obscene gesture in Alvaro’s counting scheme. Nonetheless we agree on most matters, including the Nobel Committee’s latest choice of physics laureates. Most scientific discoveries are inevitable; that of gravitational waves was not.